Roational Motion Physics
- Rotational Motion Physics Lab
- Rotational Motion Definition
- Rotational Motion Physics Problems
- Rotational Motion Physics Wallah
- Rotational Motion Physics Formulas
Torque
1. A beam 140 cm in length. There are three forces acts on the beam, F1 = 20 N, F2 = 10 N, and F3 = 40 N with direction and position as shown in the figure below. What is the torque causes the beam rotates about the center of mass of the beam?
- Kinematics is the description of motion. The kinematics of rotational motion describes the relationships among rotation angle, angular velocity, angular acceleration, and time. Let us start by finding an equation relating,. To determine this equation, we recall a familiar kinematic equation for translational, or straight-line, motion.
- In rotational motion, the particles of the rigid body follow a circular path around the rotational axis, the rotational axis could be fixed or it could be unfixed. The example for fixed axis rotational motion is the rotation of a fan, in which each particle on the blade is following a circular path around the axle of the motor of the fan.
¹Physics types usually describe rotational speed, ω, in terms of the number of 'radians' turned in a unit of time.There are a little more than 6 radians in a full rotation (2π radians, to be exact). When a direction is assigned to rotational speed, we call it rotational velocityty.).Rotational velocity is a vector whose magnitude is the rotational speed.
Known :
The center of mass located at the center of the beam.
Length of beam (l) = 140 cm = 1.4 meters
Force 1 (F1) = 20 N, the lever arm 1 (l1) = 70 cm = 0.7 meters
Force 2 (F2) = 10 N, the lever arm 2 (l2) = 100 cm – 70 cm = 30 cm = 0.3 meters
Force 3 (F3) = 40 N, the lever arm 3 (l3) = 70 cm = 0.7 meters
Wanted : The magnitude of torque
Solution :
The torque 1 rotates beam clockwise, so assigned a negative sign to the torque 1.
τ1 = F1 l1 = (20 N)(0.7 m) = -14 N m
The torque 2 rotates beam counterclockwise, so assigned a positive sign to the torque 2.
τ2 = F2 l2 = (10 N)(0.3 m) = 3 N m
The torque 3 rotates beam clockwise, so assigned a positive sign to the torque 3.
τ3 = F3 l3 = (40 N)(0.7 m) = -28 N m
The net torque :
Στ = -14 Nm + 3 Nm – 28 Nm = – 42 Nm + 3 Nm = -39 Nm
The magnitude of the torque is 39 N m. The direction of rotation of the beam clockwise, so assigned a negative sign.
2. What is the net torque acts on the beam The axis of rotation at point D. (sin 53o = 0.8)
Known :
The axis of rotation at point D
F1 = 10 N and l1 = r1 sin θ = (40 cm)(sin 53o) = (0.4 m)(0.8) = 0.32 meters
F2 = 10√2 N and l2 = r2 sin θ = (20 cm)(sin 45o) = (0.2 m)(0.5√2) = 0.1√2 meters
F3 = 20 N and l3 = r1 sin θ = (10 cm)(sin 90o) = (0.1 m)(1) = 0.1 meters
Wanted : The net torque
Solution :
τ1 = F1 l1 = (10 N)(0.32 m) = 3.2 Nm
(The torque 1 rotates beam counterclockwise so we assign positive sign to the torque 1)
τ2 = F2 l2 = (10√2 N)( 0.1√2 m) = -2 Nm
(The torque 2 rotates beam clockwise so we assign negative sign to the torque 2)
τ3 = F2 l2 = (20 N)(0.1 m) = 2 Nm
(The torque 3 rotates beam counterclockwise so we assign positive sign to the torque 3)
The net torque :
Στ = τ1 – τ1 + τ3
Στ = 3.2 Nm – 2 Nm + 2 Nm
Στ = 3.2 Nm
3. What is the net torque if the axis of rotation at point D. (sin 53o = 0.8)
Known :
The axis of rotation at point D.
Distance between F1 and the axis of rotation (rAD) = 40 cm = 0.4 m
Distance between F2 and the axis of rotation (rBD) = 20 cm = 0.2 m
Distance between F3 and the axis of rotation (rCD) = 10 cm = 0.1 m
F1 = 10 Newton
F2 = 10√2 Newton
F3 = 20 Newton
Sin 53o = 0.8
Wanted : The net torque
Solution :
The moment of the force 1
Στ1 = (F1)(rAD sin 53o) = (10 N)(0.4 m)(0.8) = 3.2 N.m
(The torque 1 rotates beam counterclockwise so we assign positive sign to the torque 1)
The moment of the force 2
Στ2 = (F2)(rBD sin 45o) = (10√2 N)(0.2 m)(0.5√2) = -2 N.m
(The torque 2 rotates beam clockwise so we assign negative sign to the torque 2)
The moment of the force 3
Στ3 = (F3)(rCD sin 90o) = (20 N)(0.1 m)(1) = 2 N.m
(The torque 2 rotates beam counterclockwise so we assign positive sign to the torque 3)
The net torque :
Στ = Στ1 + Στ2 + Στ3
Στ = 3.2 – 2 + 2
Στ = 3.2 Newton meter
The moment of inertia
4. Length of wire = 12 m, l1 = 4 m. Ignore wire’s mass. What is the moment of inertia of the system.
Known :
Mass of A (mA) = 0.2 kg
Mass of B (mB) = 0.6 kg
Distance between A and the axis of rotation (rA) = 4 meters
Distance between B and the axis of rotation (rB) = 12 – 4 = 8 meters
Wanted : The moment of inertia of the system
Solution :
The moment of inertia of A
IA = (mA)(rA2) = (0.2)(4)2 = (0.2)(16) = 3.2 kg m2
The moment of inertia of B
IB = (mB)(rB2) = (0.6)(8)2 = (0.6)(64) = 38.4 kg m2
The moment of inertia of the system :
I = IA + IB = 3.2 + 38.4 = 41.6 kg m2

5. A 6-N force is applied to a cord wrapped around a pulley of mass M = 5 kg and radius R = 20 cm. What is the angular acceleration of the pulley. The pulley is a uniform solid cylinder.
Known :
Force (F) = 6 Newton
Mass (M) = 5 kg
Radius (R) = 20 cm = 20/100 m = 0.2 m
Wanted :Angular acceleration (α)
Solution :
The moment of the force :
τ = F R = (6 Newton)(0.2 meters) = 1.2 N m
The moment of inertia for solid cylinder :
I = 1/2 M R2
I = 1/2 (5 kg)(0.2 m)2
I = 1/2 (5 kg)(0.04 m2)
I = 1/2 (0.2)
I = 0.1 kg m2.
The angular acceleration :
τ = I α
α = τ / I = 1.2 / 0.1 = 12 rad s-2
6. A block of mass = 4 kg hanging from a cord wrapped around a pulley of mass = 8 kg and radius R = 10 cm. Acceleration due to gravity is 10 ms-2 . What is the linear acceleration of the block? The pulley is a uniform solid cylinder.
Known :
Mass of pulley (m) = 8 kg
Radius of pulley (r) = 10 cm = 0.1 m
Mass of block (m) = 4 kg
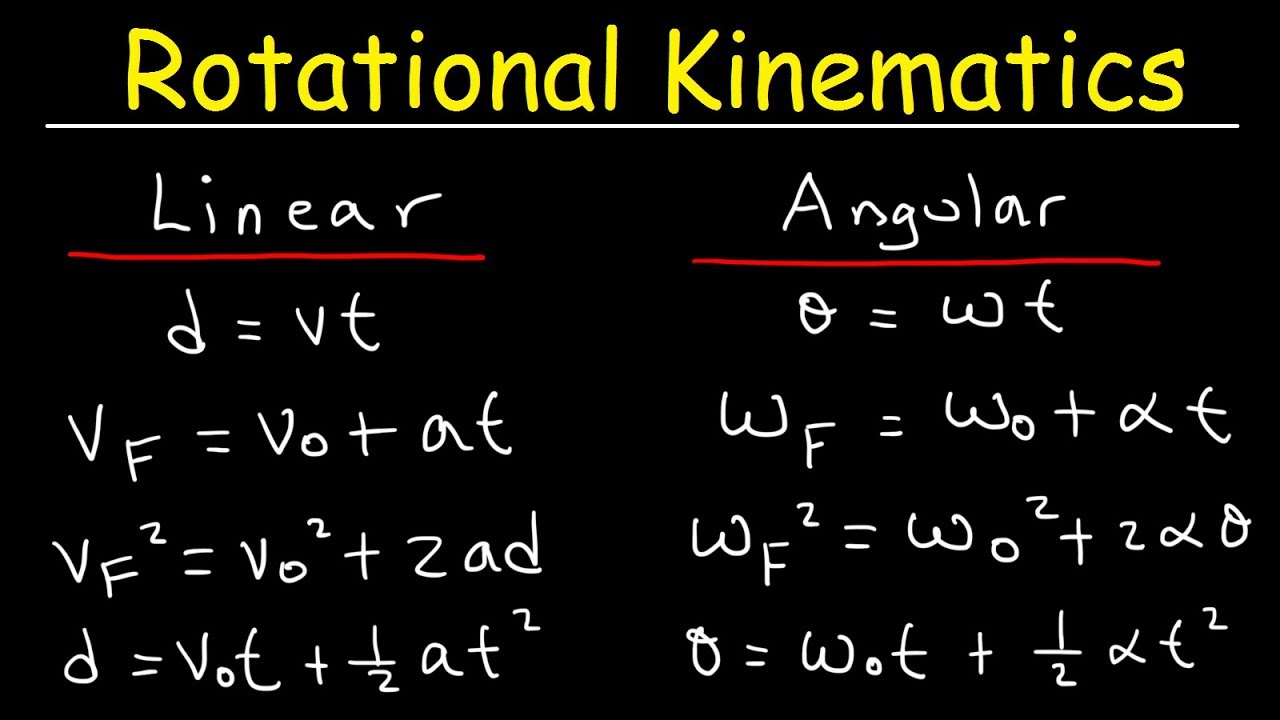
Acceleration due to gravity (g) = 10 m/s2
Weight (w) = m g = (4 kg)(10 m/s2) = 40 kg m/s2 = 40 Newton
Wanted : The free fall acceleration of the block
Solution :
The moment of inertia of the solid cylinder :
I = 1/2 M R2 = 1/2 (8 kg)(0.1 m)2 = (4 kg)(0.01 m2) = 0.04 kg m2
The moment of the force :
τ = F r = (40 N)(0.1 m) = 4 Nm
The angular acceleration :
Στ = I α
4 = 0.04 α
α = 4 / 0.04 = 100
The linear acceleration :
a = r α = (0.1)(100) = 10 m/s2
7. A block with mass of m hanging from a cord wrapped around a pulley. If the free fall acceleration of the block is a m/s2, what is the moment of inertia of the pulley..
Known :
weight = w = m g
Lever arm = R
The angular acceleration = α
The free fall acceleration of the block = a ms-2
Wanted: The moment of inertia of the pulley (I)
Solution :
The connection between the linear acceleration and the angular acceleration :
a = R α
α = a / R
The moment of inertia :
τ = I α
I = τ : α = τ : a / R = τ (R / a) = τ R a-1
The angular momentum
8. A 0.2-gram particle moves in a circle at a constant speed of 10 m/s. The radius of the circle is 3 cm. What is the angular momentum of the particle?
Known :
Mass of particle (m) = 0.2 gram = 2 x 10-4 kg
Angular speed (ω) = 10 rad s-1
Radius (r) = 3 cm = 3 x 10-2 meters
Wanted : The angular momentum of the particle
Solution :
The equation of the angular momentum :
L = I ω
I = the angular momentum, I = the moment of inertia, ω = the angular speed
The moment of inertia (for particle) :
I = m r2 = (2 x 10-4 )(3 x 10-2)2 = (2 x 10-4 )(9 x 10-4) = 18 x 10-8
The angular momentum :
L = I ω = (18 x 10-8)(10 rad s-1) = 18 x 10-7 kg m2 s-1
What is Torque?
Torque is a measure of how much a force acting on an object causes that object to rotate. The object rotates about an axis, which we will call the pivot point, and will label 'O'. We will call the force 'F'. The distance from the pivot point to the point where the force acts is called the moment arm, and is denoted by 'r'. Note that this distance, 'r', is also a vector, and points from the axis of rotation to the point where the force acts. (Refer to Figure 1 for a pictoral representation of these definitions.)
Torque is defined as (Gamma = r times F = rF sin (theta)).
In other words, torque is the cross product between the distance vector (the distance from the pivot point to the point where force is applied) and the force vector, 'a' being the angle between r and F.
The cross product, also called the vector product, is an operation on two vectors. The cross product of two vectors produces a third vector which is perpendicular to the plane in which the first two lie. That is, for the cross of two vectors, A and B, we place A and B so that their tails are at a common point. Then, their cross product, A x B, gives a third vector, say C, whose tail is also at the same point as those of A and B. The vector C points in a direction perpendicular (or normal) to both A and B. The direction of C depends on the Right Hand Rule.
If we let the angle between A and B be , then the cross product of A and B can be expressed as
(A times B = A B sin(theta))
If the components for vectors A and B are known, then we can express the components of their cross product, (C = A times B) in the following way
(C_x = A_yB_z - A_zB_y)
(C_y = A_zB_x - A_xB_z)
(C_z = A_xB_y - A_yB_x)
Further, if you are familiar with determinants, (A times B), is
(A times B = Biggr begin {matrix} i quad j quad k A_x ; A_y ; A_z B_x ; B_y ; B_z end{matrix} Biggr )
Comparing Figures CP1 and CP2, we notice that
(A times B = - B times A)
A very nice simulation which allows you to investigate the properties of the cross product is available by clicking HERE. Use the 'back' button to return to this place.
Using the right hand rule, we can find the direction of the torque vector. If we put our fingers in the direction of r, and curl them to the direction of F, then the thumb points in the direction of the torque vector.
Question
Which direction is the torque in this diagram, with respect to the pivot point labelled O?
Solution
Here we are assuming that the force, F, and moment arm, r vectors were originally placed 'head-to-head' (that is, F was pointing to the arrowhead of r, not at its pivot point). This is shown in Figure RHR 1. However, by translating the force vector to its position in Figure RHR 2, the use of the Right Hand Rule becomes more obvious.
Without this clarification, it is possible to interpret Figure RHR 2 as having the force vector going through the pivot point, in which case there would be no torque. This is due to the definition of the moment arm, which is the distance between the pivot point and the point where the force acts. If the force acts right on the pivot point, then r = 0, so there would be no torque. (Having a moment arm of zero would be like trying to open a door by pushing on its hinges; nothing happens because no torque has resulted from the applied force.)
Recall the use of the Right Hand Rule in torque calculations. Fingers are to point in the direction of the first vector, and are curled towards the second vector. In this case, torque is the cross product of the moment arm and torque. So the fingers would point to in the same direction as the moment arm, and are curled to the direction of the force (clockwise). The direction of your thumb is the direction of torque; in this case, torque is into the screen.
We can represent 'into' and 'out of' using symbols when drawing three-dimensional diagrams. The symbol for 'into' is (it's supposed to be the tail of an arrow), and for 'out of' is (this is the tip of the arrowhead).
Rotational Motion Physics Lab
Imagine pushing a door to open it. The force of your push (F) causes the door to rotate about its hinges (the pivot point, O). How hard you need to push depends on the distance you are from the hinges (r) (and several other things, but let's ignore them now). The closer you are to the hinges (i.e. the smaller r is), the harder it is to push. This is what happens when you try to push open a door on the wrong side. The torque you created on the door is smaller than it would have been had you pushed the correct side (away from its hinges).
Note that the force applied, F, and the moment arm, r, are independent of the object. Furthermore, a force applied at the pivot point will cause no torque since the moment arm would be zero (r = 0).
Rotational Motion Definition
Another way of expressing the above equation is that torque is the product of the magnitude of the force and the perpendicular distance from the force to the axis of rotation (i.e. the pivot point).
Let the force acting on an object be broken up into its tangential ((F_{tan})) and radial ((F_{rad})) components (see Figure 2). (Note that the tangential component is perpendicular to the moment arm, while the radial component is parallel to the moment arm.) The radial component of the force has no contribution to the torque because it passes through the pivot point. So, it is only the tangential component of the force which affects torque (since it is perpendicular to the line between the point of action of the force and the pivot point).
There may be more than one force acting on an object, and each of these forces may act on different point on the object. Then, each force will cause a torque. The net torque is the sum of the individual torques.
Rotational Equilibrium is analogous to translational equilibrium, where the sum of the forces are equal to zero. In rotational equilibrium, the sum of the torques is equal to zero. In other words, there is no net torque on the object.
(sum tau = 0)
Rotational Motion Physics Problems
Note that the SI units of torque is a Newton-metre, which is also a way of expressing a Joule (the unit for energy). However, torque is not energy. So, to avoid confusion, we will use the units N.m, and not J. The distinction arises because energy is a scalar quanitity, whereas torque is a vector.
Here is a useful and interesting interactive activity on rotational equilibrium. Use the 'BACK' button to return to this place. Click HERE for the activity.
Torque and Angular Acceleration
Rotational Motion Physics Wallah
In this section, we will develop the relationship between torque and angular acceleration. You will need to have a basic understanding of moments of inertia for this section.
Moment of inertia is the rotational analogue to mass. Review the definitions as explained in your text book.
The following table contains moments of inertia for various common bodies. The 'M' in each case is the total mass of the object.
Rotational Motion Physics Formulas
| Shape | Axis | Equation | Image |
|---|---|---|---|
| Slender Rod | through center | (I = frac {1}{12} cdot M cdot L^2) | |
| Slender Rod | through end | (I = frac {1}{3} cdot M cdot L^2) | |
| Rectangular Plane | through center | (I = frac {1}{12} cdot M cdot (a^2 + b^2)) | |
| Rectangular Plane | along edge | (I = frac {1}{3} cdot M cdot a^2) | |
| Sphere | thin-walled hollow | (I = frac {2}{3} cdot M cdot R^2) | |
| Sphere | solid | (I = frac {2}{5} cdot M cdot R^2) | |
| Cylinder | hollow | (I = frac {1}{2} cdot M cdot (R_ {1^2} + R_ {o^2})) | |
| Cylinder | solid | (I = frac {1}{2} cdot M cdot R^2) | |
| Cylinder | thin-walled hollow | (I = M cdot R^2) |
Imagine a force F acting on some object at a distance r from its axis of rotation. We can break up the force into tangential ((F_{tan})), radial ((F_{rad})) (see Figure 3). (This is assuming a two-dimensional scenario. For three dimensions -- a more realistic, but also more complicated situation -- we have three components of force: the tangential component (F_{tan}), the radial component (F_{rad}) and the z-component (F_z). All components of force are mutually perpendicular, or normal.)
From Newton's Second Law, (F_{tan} = m a_{tan})
However, we know that angular acceleration, (alpha) , and the tangential acceleration atan are related by:
(a_{tan} = r alpha)
Then,
(F_{tan} = m r^ alpha)
If we multiply both sides by r (the moment arm), the equation becomes
(F_{tan} r = m r^{2 alpha})
Note that the radial component of the force goes through the axis of rotation, and so has no contribution to torque. The left hand side of the equation is torque. For a whole object, there may be many torques. So the sum of the torques is equal to the moment of inertia (of a particle mass, which is the assumption in this derivation), (I = m r^2) multiplied by the angular acceleration, (alpha).
(sum tau = Icdot alpha)
If we make an analogy between translational and rotational motion, then this relation between torque and angular acceleration is analogous to the Newton's Second Law. Namely, taking torque to be analogous to force, moment of inertia analogous to mass, and angular acceleration analogous to acceleration, then we have an equation very much like the Second Law.
Example Problem: The Swinging Door
Question
In a hurry to catch a cab, you rush through a frictionless swinging door and onto the sidewalk. The force you extered on the door was 50N, applied perpendicular to the plane of the door. The door is 1.0m wide. Assuming that you pushed the door at its edge, what was the torque on the swinging door (taking the hinge as the pivot point)?
Hints
- Where is the pivot point?
- What was the force applied?
- How far from the pivot point was the force applied?
- What was the angle between the door and the direction of force?
The pivot point is at the hinges of the door, opposite to where you were pushing the door. The force you used was 50N, at a distance 1.0m from the pivot point. You hit the door perpendicular to its plane, so the angle between the door and the direction of force was 90 degrees.
Since
(tau = r times F = r F sin (theta))
then the torque on the door was:
(tau = (1.0m) (50N) sin(90))
(tau = 50 N m)
Note that this is only the magnitude of the torque; to complete the answer, we need to find the direction of torque. Using the right hand rule, we see that the direction of torque is out of the screen.